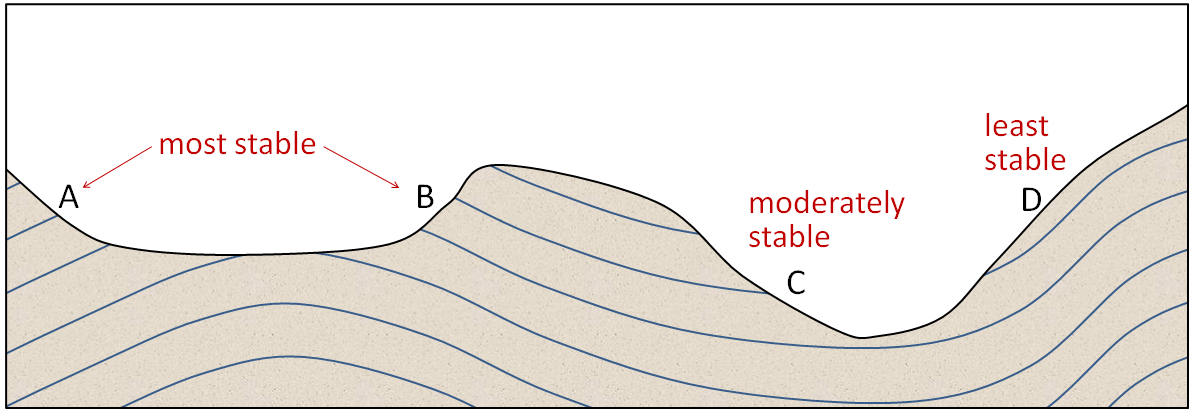
A slope refers to the sloped boundary exterior amongst air and the body of a fortification like the highways, cut or fill, earth dams, levees and also the river training works. The constancy of the slope is a very critical aspect in the civil engineering practice. A properly common engineering breakdown of slope is gliding of a ridge or cutting. The feature leading to instability can normally be categorized into:
- Those causing augmented stress and
- Those leading to a decrease in strength.
Various factors leading to augmented stress are:
a) Amplified unit mass of soil by dampening
b) Augmented extra loads (the shifting loads, buildings etc.)
c) Steepened slopes moreover by excavation or by the process of erosion
d) shock loads
loss of strength may happen because of
a) Vibration and tremors
b) Upsurge in humidity content
c) Cold and defrosting action
d) Upsurge in pore pressure
e) Forfeiture of cementing pressure
Thusly a sensible plan of the slant of these structures is exceptionally basic since an auxiliary failure may prompt to loss of human life and cash. The regular technique for a slant steadiness examination of characteristic inclines and inclines framed by cutting and filling depend on restricting the equilibrium position.
In this sort of investigation, the component of safety as to the slant dependability is assessed by analyzing the state of equilibrium when nascent failure is expected along a foreordained failure plane and afterward contrasting the quality important with keep up balance with the accessible quality of the dirt. Solidness investigation of slants depend on the accompanying suppositions:
1. The problems have to be two dimensional
2. Coulomb’s hypothesis can be utilized to register shear quality and shear quality is expected as uniform along the slip surface.
3. The flownet if there should arise an occurrence of drainage can be drawn and leakage powers assessed.
The dependability investigation could be part up into two classes:
- The dependability investigation of limitless slants
- Stability analysis in case of finite slopes
Stability analysis of the infinite slope: The limit equilibrium technique is basically utilized for the examination of finite slopes. Slopes ranging to infinity do not occurs in nature. For different practical reasons, any slope of higher extent with soil conditions fundamentally same for all equal stature below the surface are called the infinite slopes.
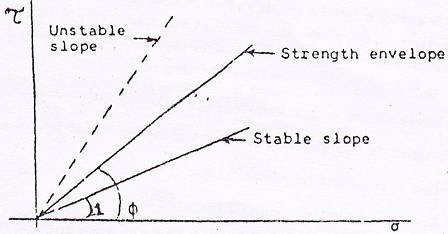
a) Infinite slopes in dry sand: The figure 1 demonstrates the failure circumstances for an infinite slope of cohesion less soil.
The issue of safety of an immeasurable slope is demarcated as the ratio of soil strength in the essential shear stress for balance. The issue of safety against sliding is expressed by:
![]()
Where ![]() =shear strength
=shear strength
![]() = mobilized shear strength due to gravity
= mobilized shear strength due to gravity
![]() = angle of internal friction
= angle of internal friction
i = inclined angle of slope
b) Infinite slope in ![]() soils: In
soils: In ![]() soil, the slope is steady as long as the slope angle i is equivalent to or minimum the angle of internal friction
soil, the slope is steady as long as the slope angle i is equivalent to or minimum the angle of internal friction ![]() . If the slope angle i, is higher than
. If the slope angle i, is higher than ![]() , the slope could be steady only upto incomplete stature called critical height is shown by:
, the slope could be steady only upto incomplete stature called critical height is shown by:
![]()
Where, c= cohesion
![]() = unit weight
= unit weight
![]() = angle of internal friction
= angle of internal friction
i = slope angle
![]() = critical height
= critical height
If the factor of safety Fc is utilized in cohesion, the equipped cohesion at depth H, given by
![]()
Then the depth H computed by utilizing mobilized cohesion Cm will not be acute. the issue of safety against stature also signifies the feature of safety with respect to cohesion Fc.
Fc is shown by
![]()
A dimensionless parameter called a stability number is normally utilized for the examination of slopes of ![]() soils and can be known by the subsequent computation
soils and can be known by the subsequent computation
![]()
Where Sn= stability number (a dimensionless quantity)
The reciprocal of stability number is called stability factor.
Fig.2 demonstrates the failure form of an infinite slope of cohesive soil.
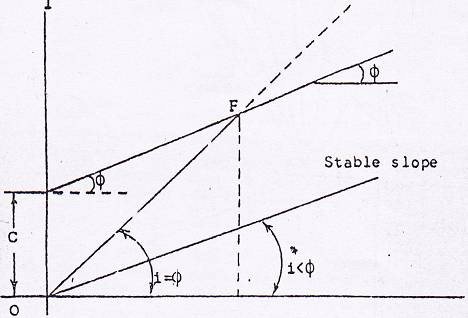
Figure 2- failure condition of infinite slope of cohesive soil
Stability analysis of finite slopes:
Failure of finite slopes happens alongside a bent surface. In stability examination of finite slopes, the real surface of rift is substituted by a curve of a round. As to the manner of breakdown, the slope might fail essentially in the following two categories:
a) The failure surface passing via the toe of the slope or beyond the toe of slope is called slope failure.
b) The rupture is deep seated and passes through the ridge retaining soil below the toe of the slant is called base failure.
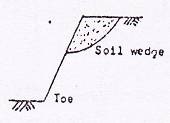 a) Slope failure above toe (or face failure)
a) Slope failure above toe (or face failure)
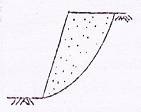 b) slope failure through toe
b) slope failure through toe
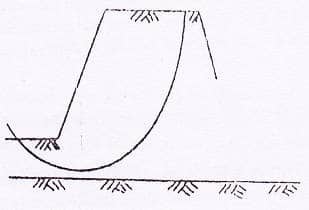 c) Base failure
c) Base failure
The base failure normally happens specifically when the soil below the embankment is weaker and more elastic than the slant creating soil itself. There are different techniques easily available for the constancy analysis nonetheless the subsequent methods are simple and extensively apploed
1. Slip circle method (Swedish circle technique)
2. Friction circle technique
Swedish circle method or method of slices: The technique accepts the surface of sliding is an curve of a circle. This was recognized by learning the failure or embankments in Sweden. (fig.4)
Analysis of the purely cohesive soils ( ![]() = 0 analysis)
= 0 analysis)
Consider a probable spherical slip surface AD (fig.4) with centre at O.
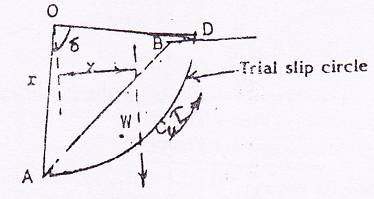
The disturbing moment of the cylinder of the soil about O= Wx
Where ‘x’ is the distance of the line of action of W from the vertical line passing through the center of rotation.
If Cu is the unit cohesion, L= length of the slip arc,
![]()
The shear resistance developed along the slip surface will be equal to Cu.L.
The resisting moment avoiding the soil from shifting is all because of friction along arc length AD which has a lever arm equivalent to radius r about O.
Resisting moment = cohesion x arc length AD x r = CuL.r
Factor of safety against sliding = (Resisting moment / Disturbing moment)
![]()
A sequence of slip circles is checked, and the lowest factor of safety is the most probable failure plane.
![]() Soil (
Soil (![]() Analysis)
Analysis)
In order to study stability of the slope of a ![]() soil, a possible slip circle is selected and separated into bands of equivalent width as revealed in fig 5 (a) and (b).
soil, a possible slip circle is selected and separated into bands of equivalent width as revealed in fig 5 (a) and (b).
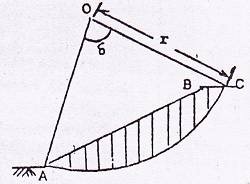
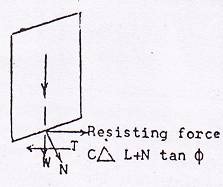
Disturbing moment about centre O = T x r
Total driving moment = ![]()
Where ![]() = algebraic sum of all tangential components
= algebraic sum of all tangential components
The resisting force on one strip is made up of cohesion and friction and is given by:
Resisting force= ![]()
Resisting moment on one strip= ![]()
Total resisting moment = ![]()
![]()
Where ![]() = sum of all normal components, L= arc length
= sum of all normal components, L= arc length
Factor of safety
![]()