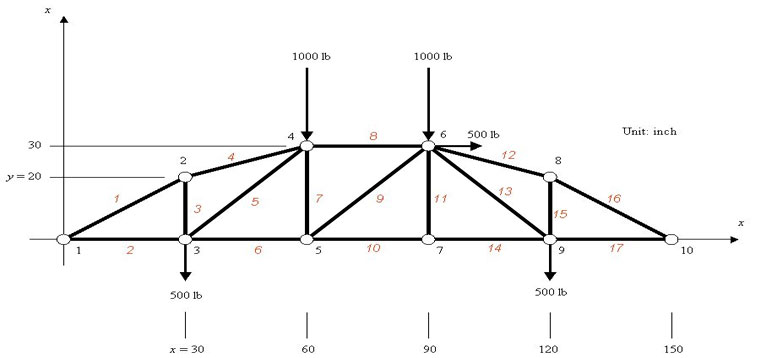
In this article, you can study truss analysis techniques with examples. Analysis of truss by the techniques of joints and by the means of section are explained in brief in the article.

We have studied about the basics of the equilibrium of bodies; now we will debate about the trusses that are basically utilized in creation of stable load-bearing structures. The instances of these are the sides of the bridges or tall tower structures or those towers that carry electricity wires. Schematic diagram of a structure on the side of a bridge is drawn and illustrated in the figure below.

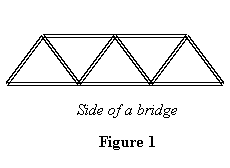
The structure as per shown above is fundamentally structure with two dimensions. It is also called plane truss. Similarly, on the other hand, a tower of microwave oven or telephones are a three-dimensional structure. Consequently, there are basically 2 types of trusses – Plane trusses just like on the sides of a bridge and another one being space trusses like the TV towers. Here, we will be studying about plane trusses in which the basis elements are joined together in the plane.

To stimulate the structure of a plane truss, we will now take a slender rod (12) amongst points 1 and 2 and join it to a attached pin joint at 1 (see figure 2).

Presently I put a pin (pin2) at point 2 at the upper end and suspend a weight W on it. The question is whether there arises the need to hold the weight by then, what other least backings would it be advisable for us to give? For rods we are to make just pin joints (We accept everything is in this plane and the structures does not topple side ways). Since bar (12) tends to turn clockwise, we stop the rightward development of point 2 by associating a bar (23) on it and afterward prevent direct 3 from moving toward the privilege by interfacing it to point 1 by another pole (13). Every one of the joints in this structure are stick rods.. Notwithstanding, in spite of this the whole structure still tends to swing to turn clockwise on the grounds that there is a torque on it because of W. To counter this, we connect a wheel on point 3 and put it on the ground. This is the absolute minimum that we require to hold the weight is place. The triangle made by poles shapes the premise of a plane truss.

Note

At this point, the question may arise as to explain need the parallel rod (13). It is due to the fact that the point 3 will then keep shifting to the right making the entire construction unhinged. Rod (13) will two forces functioning on it: one perpendicular force because of the wheel and the other one will be at the end 2. Nevertheless, these two forces will not be collinear so exclusive of the rod (13) the system shall not be in the position of the equilibrium. Commonly, in a truss all of the joint has to be associated to minimum three or at least two rods and additionally one external support as well.

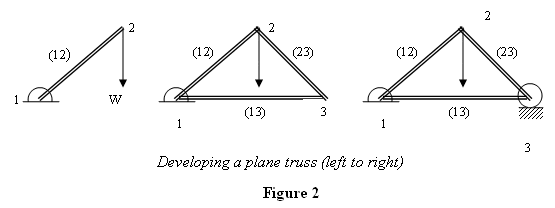
Now we will analyze forces in the construction structure that were just created. For the purpose of straightforwardness, now we will choose lengths of all rods to be equivalent. To obtain the forces I, now we will look at all the forces on every one of the pin and bargain circumstances under which the pins will be in the equilibrium position. The initial thing we mark down that every one of the rod in equilibrium when they are exposed to the influence of two forces which is exerted by the pins at their conclusions. As we had studied in the previous chapter, in circumstances like these the forces will have to be collinear and consequently along the rods only. Therefore each one of the rod is under a tensile or compaction force. As a result, rods (12), (23) and (13) experience forces as illustrated in figure 3.

We have to pay attention to the fact that we have taken all the forces to be compaction. If the actual forces are ductile in nature, the result will lead out to be negative. We will now look at pin 2. The singular forces acting on pin 2 are F₁₂ because rod (12) and F₂₃ because rod (23). Additional, it is pulled downwards due to the weight W. Therefore, forces acting on pin 2 look like illustrated in the figure 4.

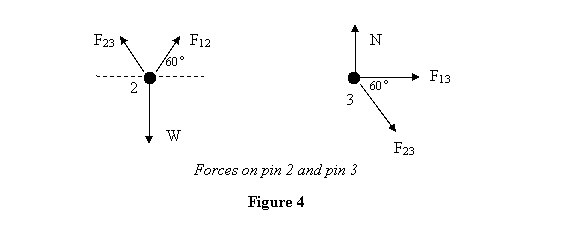
Applying equilibrium condition to pin (2) results to

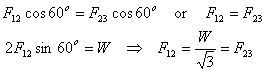
We will now examine at pin 3 (see figure 4). It is in equilibrium condition under forces F₂₃, normal reaction N and a parallel force F₁₃.

Applying equilibrium condition  yields

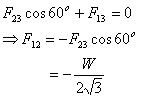
Because the direction of F₁₃ is coming out to be negative, the direction should be opposite to that assumed. Balance of forces in the vertical direction provides

Consequently, we see that the weight is retained with these three rods. The construction structure is determinate and it retains the weight in place as well.

Even if we substitute the pin joints by a minor plate (also called the gusset plate) with at least two or three pins in these, the study relics pretty much the same as previous owing to the fact that the pins are so near organized that they barely generate any moment about the joints. Even in those case when if the rods are fused together at the joints, to a higher extent of accurateness majority of the force is undertaken longitudinally on the rods, even though some very minor (insignificant) moment is formed by the joints and might be feasible bending of the rods.

From this point, we are all ready to construct a truss and examine it as well. Now, we are going to construct it by augmenting higher and more of triangles fused together. Now, consequently when the triangles are added, the member of joints j and additionally the number of members (rods) m are related shown below:

m = 2j – 3

After this, it will make a truss statically determinate. This is easily comprehendible as follows. First of all, we will consider the whole truss as one system. If those case where it has to be statically determinate, there has to be just three unidentified forces on it due to the fact for forces in a plane there are at least three equilibrium conditions. Attaching one of its ends a pin joint and keeping the other one on the roller does that (roller also provides the augmented benefit that it can aid in regulating any alteration in the interval of a member because of different causes like deformations). If we want to compute these outside forces and the force in every member of the truss, the complete number of unknowns converts m + 3. We resolve for these unknowns by writing equality circumstances for each pin; there has to be 2j such equations. For the system to be completely determinate we need to have m + 3 = 2j , which is the condition as state above. If the case arise where we need to augment any more members, these are dismissed. On the other side, lesser number of members would create the truss unbalanced and it will breakdown when loaded. This will occur since the truss will not be capable to deliver the prerequisite number of forces for all equilibrium circumstances to be fulfilled. Statically determinate trusses are also called simple trusses.

Exercise 1: As per illustrated in the figure 5 are three usually utilized trusses on the sides of bridges. Demonstrate that all three of them are simple trusses.

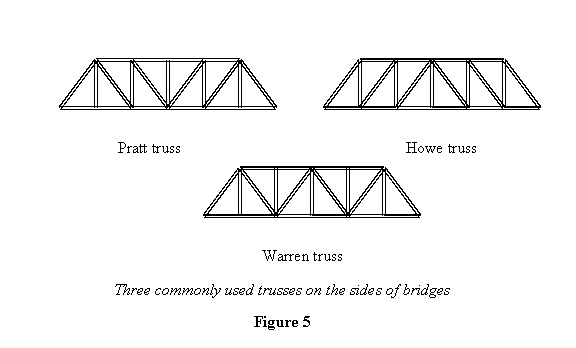
The questions may arise as why we keep the trusses on the bridges.. As our advanced examination will display they allocate the load over all elements and thus building the bridge stronger and more robust.

We will now work towards the process to get the forces made in numerous arms of a truss when it is laden outwardly. This is carried out under the given expectations:

1. In those cases, where the middle line of the members of a truss meet at a given point, that point will be assumed as a pin joint. It is a very critical assumption since we have seen previously while presenting a truss (triangle with pin joint), the load is transported on to another member of the trusses so that forces will remain fundamentally collinear with the member.
2. All of the external loads are exerted on pin connections.
3. All members’ weight is correspondingly distributed on connecting pins.

There are basically two approaches of shaping forces in the members of a truss – Technique of joints and system of sections. We initiate with the technique of joints:

Truss Analysis- Method of Joints

In this technique of joints, we shall analyze the equilibrium of the pin at the joints. Because of the facts that the forces are synchronized at the pin, it is exclusive of moment equation and just two equations for equilibrium viz.  . Consequently, we will begin our analysis at a point where one identified load and at maximum two unidentified forces are there. The mass of all the members is allocated into two halves and that is reinforced by each pin. To a degree, we have by this time referred to this technique while presenting trusses. We will now illustrate it with help of examples.

Example 1: we will take truss ABCDEF as illustrated in figure 6 and load it at the specific given point E by 5000N. The stretch of small members of the truss is 4m and the length of the diagonal members is  m. we will now ascertain the forces in all of the members of this truss taking after the assumption that they are weightless.

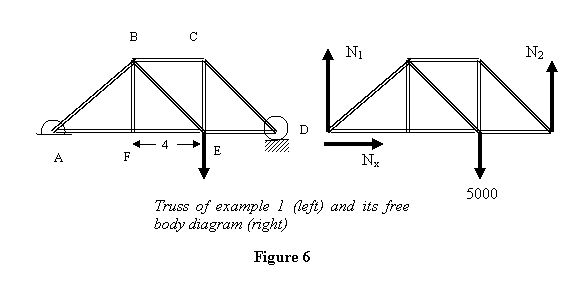
Thereafter, now we will take each point to be a pin joint and begin corresponding forces on all of the existing pins. Due to the fact that the pin E has an exterior load of 5000N one might need to commence from there. Though, E point consists of more than 2 unidentified forces so we will not be able to begin at E. We thus first give the truss as a entire and catch reactions of ground at the given points A and D owing to the fact that then at points A and D their will persist just two unidentified forces. The horizontal reaction Nx at point A is nil due to the fact that there is no exterior horizontal force on the system. To ascertain N₂ I take moment about A to get

Which through equation results to

In the techniques of the joints, we will now commence at pin A and balance the numerous forces. We by now expect the course and demonstration their almost at A (figure 7). All the approaches that the diagonals make are 45° .

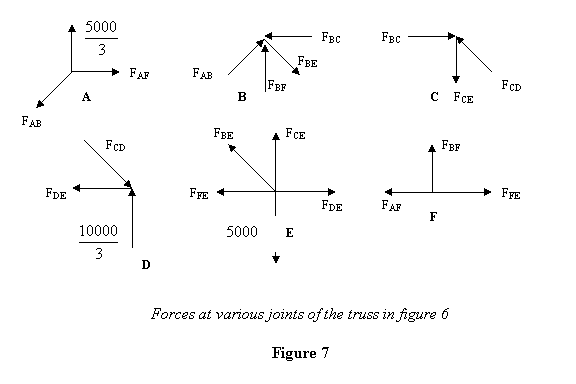
The only equations we now have to be concerned about are the force balance equations.

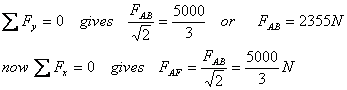
We have to make sure that the force on the member AB and AF working to be contrary to the forces on the pin ( Newton ‘s III^rd law). Consequently, force on member AB is compaction (pushes pin A away) however that on AF is ductile (pulls A towards the aforementioned).

Now we will undertake the joint F where force AF is recognized and two forces BF and FE are unidentified. For pint F

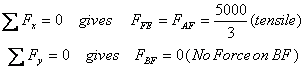
Now we will analyze the point B because now there are only two unidentified forces there. At point B

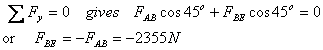
Negative sign indicates that while we have revealed F_BE to be compressive, it is really tensile.

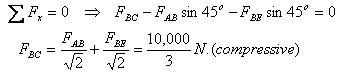
At this point, we will now contemplate point C and equilibrium the forces there. I have previously projected the course of the forces and presented F_CE to be ductile whereas F_CD to be compressive

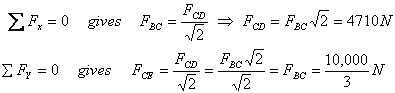
From there, we will go to pin D where the normal reaction is  N and balance forces there.

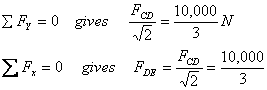
Therefore, forces in numerous adherents of the truss have been ascertained. They are

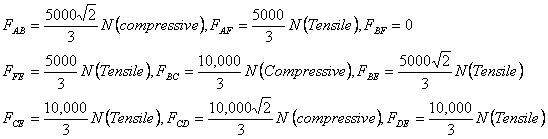
Now the question that arises is the process of how we got all the forces exclusive of utilization of equations at all joints. Remember that is how we had acquired the statical determinacy condition. We did not have to utilize all joints since previously we had preserved the structure as an unabridged and had acquired two equations from there. So one joint – in this case E – has got no need to be examined. Though, given the fact that the truss is statically determinate, all these forces have to balance at point E, where the load shall be exerted also. How the situation would vary if each member of the truss had weight. Let’s assume that every members weighs approximately 500N, then we will assume that the load is separated correspondingly among two pins supporting the member the loading of the truss would seem as set in figure 8 (loading because of the weight as shown in red). Except at points A and D the loading because the weight is 750N; at the A and D points it is 500N.

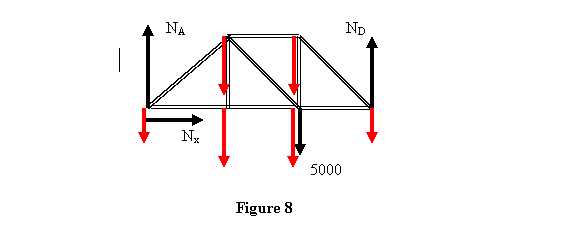
Now the exterior reaction at each end will be:

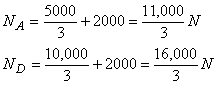
The additional 2000N could be computed from the moment equation or they can also be computed straightaway by understanding that the new augmented weight is faultlessly symmetric about the midpoint of the truss and consequently will be correspondingly separated among the two supports. For the process of balancing forces at other pins, we trail the same process as above, ensuring though that all of the pin has an external loading because of the weight of each member. We’ll solve for forces in some member of the truss. Looking at pin A, we obtain:

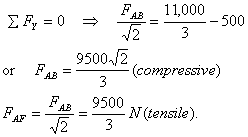
Now, we move to point F and see that the forces are

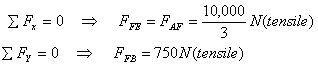
Now we can likewise solve for other pins in the truss we will complete that as an exercise for you.

After the demonstration of the method of joints, we will now shift on to look at the technique of sections that straight provides the force on a preferred member of the truss.

Truss Analysis- Method of sections

Just like the name suggests, in this technique of sections we make segments through a truss and then compute the force in the members of the truss though which the cut is created. For instance, if we take the trick we just solved in the technique of joints and create a section S₁, S₂ (see figure 9), we can then easily ascertain the forces in members BC, BE and FE by bearing in mind the equilibrium of the segment to the left or the right of the unit.

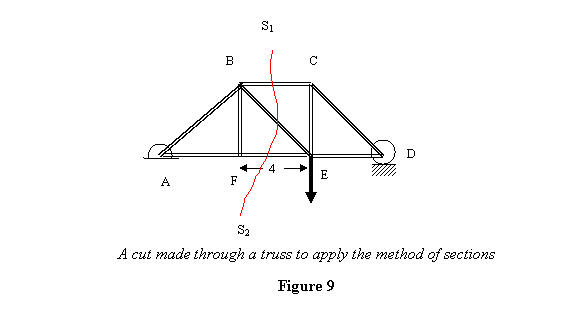
We will now show this. Similar to the method of joints, we initiate by first of all shaping the reactions at the exterior support of the truss by considering it as a completely inflexible body. In the current case scenario, it provides N at D and  N at A. Now we will assume the section of the truss on the left (see figure 10).

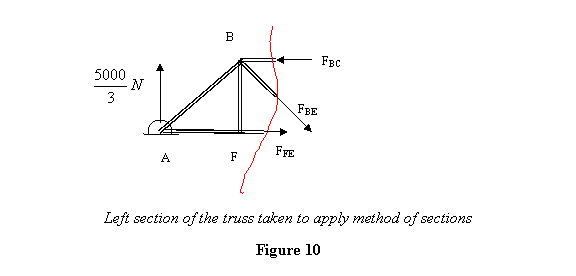
Because the complete section is in equilibrium,  . Note down that we are now utilizing all three equations for equilibrium because the forces in all of the members are not simultaneous. The course of force in each member, we can now ascertain it by inspection. Consequently, the force in the section of members BE must be pointing down due to the fact that there is no other member that can provide a descending force to counterbalance  N reaction at A. This obviously demonstrates that F BE is tensile. Correspondingly, to counter the torque about B created by  N force at A, the force on FE will also has to be from F to E. As a result, this force is also ductile. If we next contemplate the balance of torque about A,  N and F_FE do not provide any torque about A. So to counter torque created by F_BE , the force on BC must act towards B, thus then making the force compressive.

We will now calculate individual forces. F_FE is the simplest to compute. For it we take the moment about B. This provides

4 ×  = 4 × F FE

F FE =  N

Now, we calculate F_BE . For this, we use the equation  . It gives

Lastly, for calculation of F_BC , we can utilize either the equation  about A or 

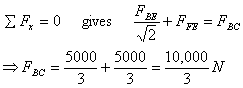
Finally we have ascertained the forces in these three members straight without computing forces going from one joint to next joint and have preserved ample amount of time and effort during the whole process. The forces on the right segment shall be contrary to those on the left segments at points from side to side which the segment is cut. This could be utilized to check the result and it will be an exercise for you.

After this diagram we will show the different steps that are undertaken in order to solve for forces in members of a truss by the technique of sections:

1. first of all, create a cut to divide the truss into segment, passing the cut through members where the force is desirable.

2. Create the cut through three member of a truss due to the fact that with three equilibrium equations viz.  we can resolve for a maximum of three forces.

3. Exert equilibrium conditions and resolve for the preferred forces.

In utilization of the method of sections, ingenuity lies in creating a proper. The technique after a way of directly computing required force circumventing the hard work included in utilizing the method of joints where we will have to solve for each joint.

Share it on: